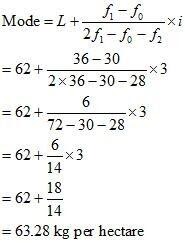NCERT QUESTIONS AND ANSWERS

1.Which average would be suitable in the following cases?

(i) Average size of readymade garments.

Ans. The demand for the average size of any readymade garment is the maximum. As, the modal value represents the value with the highest frequency, so the number of the average size to be produced is given by the Modal value

(ii) Average intelligence of students in a class.

Ans. Median will be the best measure for calculating the average intelligence of students in a class. It is the value that divides the series into two equal parts. So, number of students below and above the average intelligence can easily be estimated by median.

(iii) Average production in a factory per shift.

Ans. It is advisable to use mean for calculating the average production in a factory per shift. The average production is best calculated by arithmetic mean.

(iv) Average wage in an industrial concern.

Ans. Mean will be the most suitable measure. It is calculated by dividing the sum of wages of all the labour by the total number of labours in the industry.

(v) When the sum of absolute deviations from average is least.

Ans. When the sum of absolute deviations from average is the least, then mean could be used to calculate the average. This is an important mathematical property of arithmetic mean. The algebraic sum of the deviations of a set of n values from A.M. is 0.

(vi) When quantities of the variable are in ratios.

Ans. Median will be the most suitable measure in case the variables are in ratios. It is least affected by the extreme values

(vii)In case of open-ended frequency distribution.

Ans.  In case of open ended frequency distribution, Median is the most suitable measure as it can be easily computed. Moreover, the median value can be estimated even in case of incomplete statistical series.

2.Indicate the most appropriate alternative from the multiple choices

provided against each question.

(i) The most suitable average for qualitative measurement is

(a) arithmetic mean

(b) median

(c) mode

(d) geometric mean

(e) none of the above

(ii) Which average is affected most by the presence of extreme items?

(a) median

(b) mode

(c) arithmetic mean

(d) none of the above

(iii) The algebraic sum of deviation of a set of n values from A.M. is

(a) n

(b) 0

(c) 1

(d) none of the above

[Ans. (i) b (ii) c (iii) b]

3.Comment whether the following statements are true or false.

(i) The sum of deviation of items from median is zero.

(ii) An average alone is not enough to compare series.

(iii) Arithmetic mean is a positional value.

(iv) Upper quartile is the lowest value of top 25% of items.

(v) Median is unduly affected by extreme observations.

[Ans. (i) False (ii) True (iii) False (iv) True (v) False]

4.If the arithmetic mean of the data given below is 28, find (a) the missing frequency, and (b) the median of the series:

Profit per retail shop (in Rs)	0 – 10	10 – 20	20 – 30	30 – 40	40 – 50	50 – 60
Number of retail shops	12	18	27	–	17	6

Ans. (i) Let the missing frequency be f1

Arithmetic Mean = 28

Profit per Retail Shop (in Rs)	No of Retail Shops	Mid Value
Class Interval	(f)	(m)	fm
0 – 10	12	5	60
10 – 20	18	15	270
20 – 30	27	25	675
30 – 40	f1	35	35f1
40 – 50	17	45	765
50 – 60	6	55	330

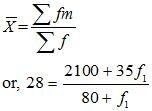
or, 2240 + 28f1 = 2100 + 35f1

or, 2240 – 2100 = 35f1 – 28f1

or, 140 = 7f1

f1 = 20

(ii)

	Class Interval	Frequency (f)	Cumulative Frequency (CF)
	0 – 10	12	12
	10 – 20	18	30
	20 – 30	27	57
	30 – 40	20	77
	40 – 50	17	94
	50 – 60	6	100
	Total

So, the Median class = Size of 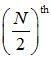  item

= 50th item

50th item lies in the 57th cumulative frequency and the corresponding class interval is 20 – 30.

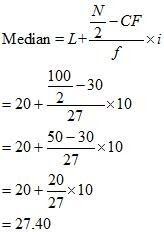
5. The following table gives the daily income of ten workers in a factory. Find the arithmetic mean.

Workers	A	B	C	D	E	F	G	H	I	J
Daily Income (in Rs)	120	150	180	200	250	300	220	350	370	260

Ans

Workers	Daily Income (in Rs) (X)
A	120
B	150
C	180
D	200
E	250
F	300
G	220
H	350
I	370
J	260
Total

N = 10

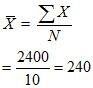
Arithmetic mean = Rs 240

 

6. Following information pertains to the daily income of 150 families. Calculate the arithmetic mean.

Income (in Rs)	Number of families
More than 75	150
More than 85	140
More than 95	115
More than 105	95
More than 115	70
More than 125	60
More than 135	40
More than 145	25

Ans.

Income	No. of families	Frequency	Mid Value	fm
Class Interval	(CF)	(f)	(m)
75 – 85	150	150 – 140 = 10	80	800
85 – 95	140	140 – 115 = 25	90	2250
95 – 105	115	115 – 95 = 20	100	2000
105 – 115	95	95 – 70 = 25	110	2750
115 – 125	70	70 – 60 = 10	120	1200
125 – 135	60	60 – 40 = 20	130	2600
135 – 145	40	40 – 25 = 15	140	2100
145 – 155	25	25	150	3750
Total

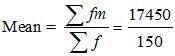
= Rs 116.33

L

7. The size of land holdings of 380 families in a village is given below. Find the median size of land holdings.Find the median size of land holdings.

Size of Land Holdings (in acres)	Less than 100	100 – 200	200 – 300	300 – 400	400 and above
Number of families	40	89	148	64

Ans

Size of Land Holdings Class Interval	No. of Families (f)	Cumulative Frequency (CF)
0 – 100	40	40
100 – 200	89	129
200 – 300	148	277
300 – 400	64	341
400 – 500	39	380
Total

So, the Median class = Size of  item = 190th item

190th item lies in the 129th cumulative frequency and the corresponding class interval is 200 – 300.

Median size of land holdings = 241.22 acres

 

8.The following series relates to the daily income of workers employed in. a firm. Compute (a) highest income of lowest 50% workers (b) minimum income earned by the top 25% workers and (c) maximum income earned by lowest 25% workers.

Daily Income (in Rs)	10 – 14	15 – 19	20 – 24	25 – 29	30 – 34	35 – 39
Number of workers	5	10	15	20	10	5

(Hint: Compute median, lower quartile and upper quartile)

9.The following series relates to the daily income of workers employed in. a firm. Compute (a) highest income of lowest 50% workers (b) minimum income earned by the top 25% workers and (c) maximum income earned by lowest 25% workers.

Daily Income (in Rs)	10 – 14	15 – 19	20 – 24	25 – 29	30 – 34	35 – 39
Number of workers	5	10	15	20	10	5

(Hint: Compute median, lower quartile and upper quartile)

Ans.

Daily Income (in Rs) Class Interval	No. of Workers (f)	Cumulative frequency (CF)
9.5 – 14.5	5	5
14.5 – 19.5	10	15
19.5 – 24.5	15	30
24.5 – 29.5	20	50
29.5 – 34.5	10	60
34.5 – 39.5	5	65

 

(a) Highest income of lowest 50% workers

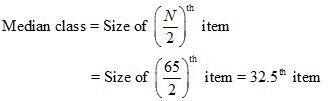
32.5th item lies in the 50th cumulative frequency and the corresponding class interval is 24.5 – 29.5.

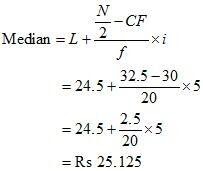
(b) Minimum income earned by top 25% workers
In order to calculate the minimum income earned by top 25% workers, we need to ascertain Q3.

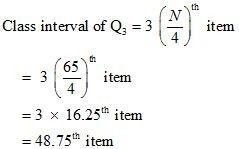
48.75th item lies in 50th item and the corresponding class interval is 24.5 – 29.5.

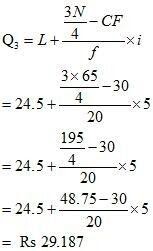
(c) Maximum  income earned by lowest 25% workers
In order to calculate the maximum income earned by lowest 25% workers, we need to ascertain Q1.

16.25th item lies in the 30th cumulative frequency and the corresponding class interval is 19.5 – 24.5

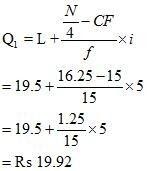
9. The following table gives production yield in kg. per hectare of wheat of 150 farms in a village. Calculate the mean, median and mode values.

Production yield (kg. per hectare)	50 – 53	53 – 56	56 – 59	59 – 62	62 – 65	65 – 68	68 – 71	71 – 74	74 – 77
Number of farms	3	8	14	30	36	28	16	10	5

Ans

(i) Mean

Production Yield	No. of farms	Mid value	A = 63.5
50 – 53	3	51.5	–12	–4	–12
53 – 56	8	54.5	–9	–3	–24
56 – 59	14	57.5	–6	–2	–28
59 – 62	30	60.5	–3	–1	–30
62 – 65	36	63.5	0	0	0
65 – 68	28	66.5	+3	+1	28
68 – 71	16	69.5	+6	+2	32
71 – 74	10	72.5	+9	+3	30
74 – 77	5	75.5	+12	+4	20
Total

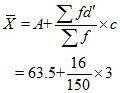
= 63.5 + 0.32

= 63.82 kg per hectare

 

(ii) Median

 

Class Interval	Frequency (f)	CF
50 – 53	3	3
53 – 56	8	11
56 – 59	14	25
59 – 62	30	55
62 – 65	36	91
65 – 68	28	119
68 – 71	16	135
71 – 74	10	145
74 – 77	5	150
Total

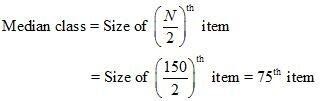
75th item lies in the 91st cumulative frequency and the corresponding class interval is 62 – 65.

(iii) Mode

 

Grouping Table
Class Interval	I	II	III	IV	V	VI
50 – 53	3
		11	22	25	52
53 – 56	8
56 – 59	14
		44
59 – 62	30
62 – 65
			44			54
65 – 68	28
68 – 71	16
		26	15	31
71 – 74	10
74 – 77	5

 

Analysis Table

 

Column	50 – 53	53 – 56	56 – 59	59 – 62	62 – 65	65 – 68	68 – 71	71 – 74	74 – 77
I					√
II					√	√
III				√	√
IV				√	√	√
V					√	√	√
VI			√	√	√
Total	–	–	1	3	6	3	1	–	–

 

Modal class = 62 – 65